Calculate the Cylinder Size
By Ernie Parker, CFPAI, CFPSD, CFPS, CFPMM, CFPMT, CFPMIP, CFPMMH, CFPMIH, CFPE
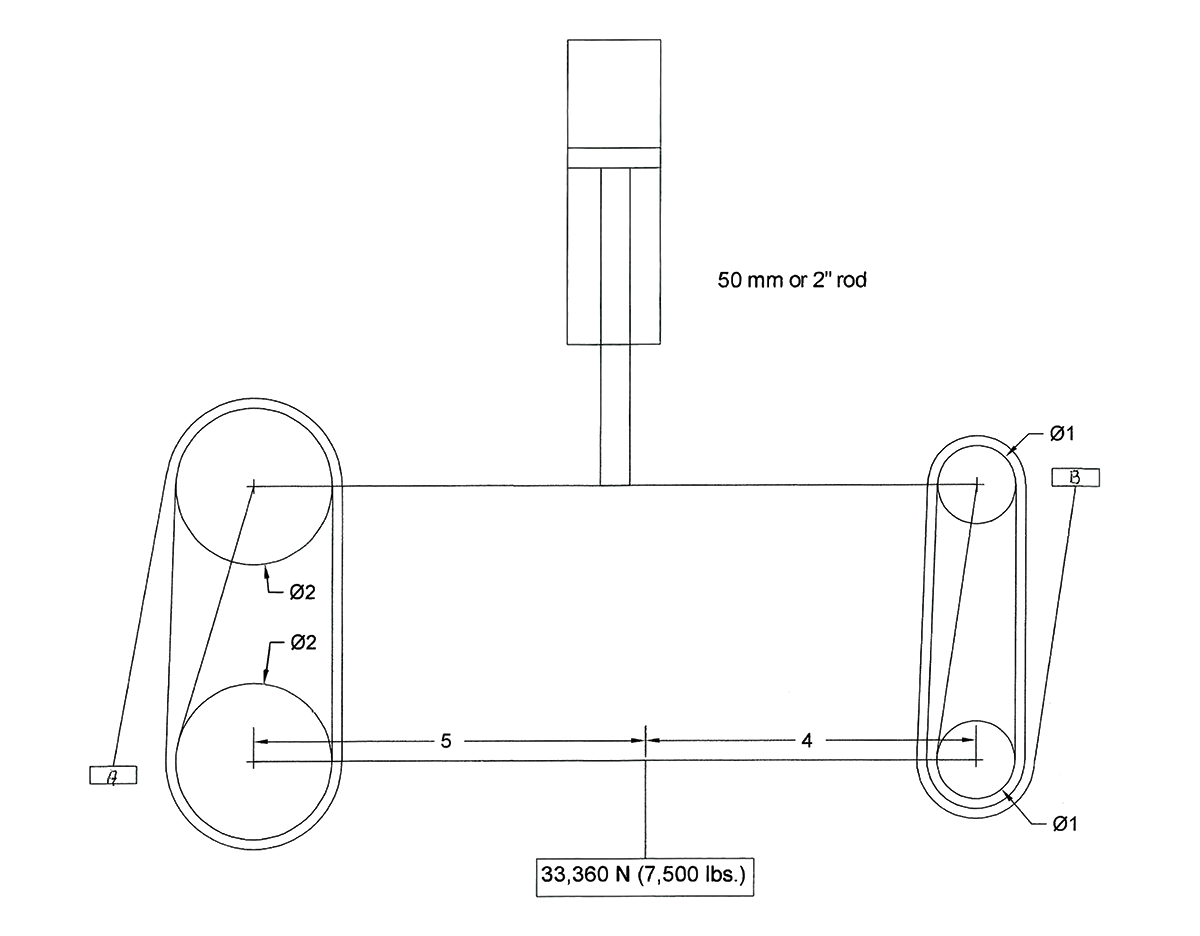
Metric Question
We have a cylinder with a 50 mm rod supporting a bar with two pulleys as shown. Calculate the standard size cylinder needed to hold the load at .71 MPa. Also, what is the force needed at the end of each cable to hold the load?
US Customary Question
We have a cylinder with a 2” rod supporting a bar with two pulleys as shown. Calculate the standard size cylinder needed to hold the load at 100 psi. Also, what is the force needed at the end of each cable to hold the load?
See the soultion
Metric Solution:
Solving for the size of the cylinder; Area of rod = 1963.5 mm², Force = 33,360 N; F= PA; 33,360/.71 = 46,985.92 mm² (area); 46,985.82 + 1963.5 (rod area) = 48,949.42 mm² needed area. Find diameter using A = D² x .7854; Sq. Root of (50,409.72/.7854) = 249.65 mm diameter Round up to 250 mm in diameter.
“A” has 4/9 of the total load. 33,360 x 4/9 = 14,826.67 N.; 14,826.67/4 cables on the movable pully = 3,706.67 N.
“B” has 5/9 of the total load. 33,360 x 5/9 =18,533.33 N.; 18,533.33 /6 cables on the movable pully = 3,088.88 N.
US Customary Solution:
7500 lbs. /100 psi = 75 sq. in. EREA needed, 75 + 3.14 sq. in. of rod = 78.14 sq. in. total. Using A = D² x .7854; Sq. root of (78.14 / .7854) = 10 in diameter.
“A” has 4/9 of the total load. 7500 x 4/9 = 3333.33 lbs. There are 4 cables on the movable pully. 3333.33 / 4 = 833.33 lbs.
“B” has 5/9 of the total load. 7500 x 5/9 = 4166.67 lbs. There are 6 cables on the movable pully. 4166.67 / 6 = 694.44 lbs.