Problem: Necessary Pressure

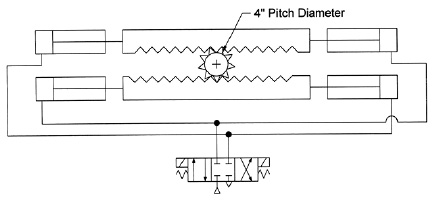
If the rotary actuator has four 4″ diameter cylinders as shown, what would be the necessary pressure to rotate the shaft with a torque of 5000 lb. in.? Assume 100% efficiency.
Also calculate the CIR (displacement).
Find Out The Solution
Given: Torque is 5,000 lb. in.
Four cylinders with a diameter of 4 inches (two pushing at one time)
Four-inch pitch diameter of pinion gear
Step 1: Find push for the cylinders: T = RP (Torque = Radius and push or pull)
Radius of the pinion gear is 2 inches.
5,000 lb. in. = 2″ x Push
P = 2,500 lbs. (for two cylinders). Note again that only two cylinders are pushing at a time.
Step 2: Find push for each cylinder: 2,500 total / 2 = 1,250 lbs. per cylinder
Step 3: Find area of 4″ cylinder: A = D² x 0.7854 A = 12.566 sq. in.
Find pressure requirements: F = PA
1250# / 12.566 = 99.47 psi or rounding up:
100 psi
Deadline past. Not available for submissions.
Winner:
Ismail Polat, CFPPS, Airline Hydraulics, Inc., Ocean View, NJ
Answered Correctly:
Raman Benipal, JEM Technical, Winnipeg, Canada
George Fling, CFPS, Dallas, TX
By Ernie Parker, AI, AJPP, AJPPCC, S, MT, MM, MIH, MIP, MMH,
Fluid Power Instructor, Hennepin Technical College, EParker@Hennepintech.edu
This teaser is printed in the Fluid Power Journal. Those who submit the correct answer before the deadline will have their names printed in the Society Page newsletter and in Fluid Power Journal. The winners will also be entered into a drawing for a special gift.