Understanding the Theoretical Force of a Cylinder

Elements of this image furnished by Adobe Stock | Evgeny
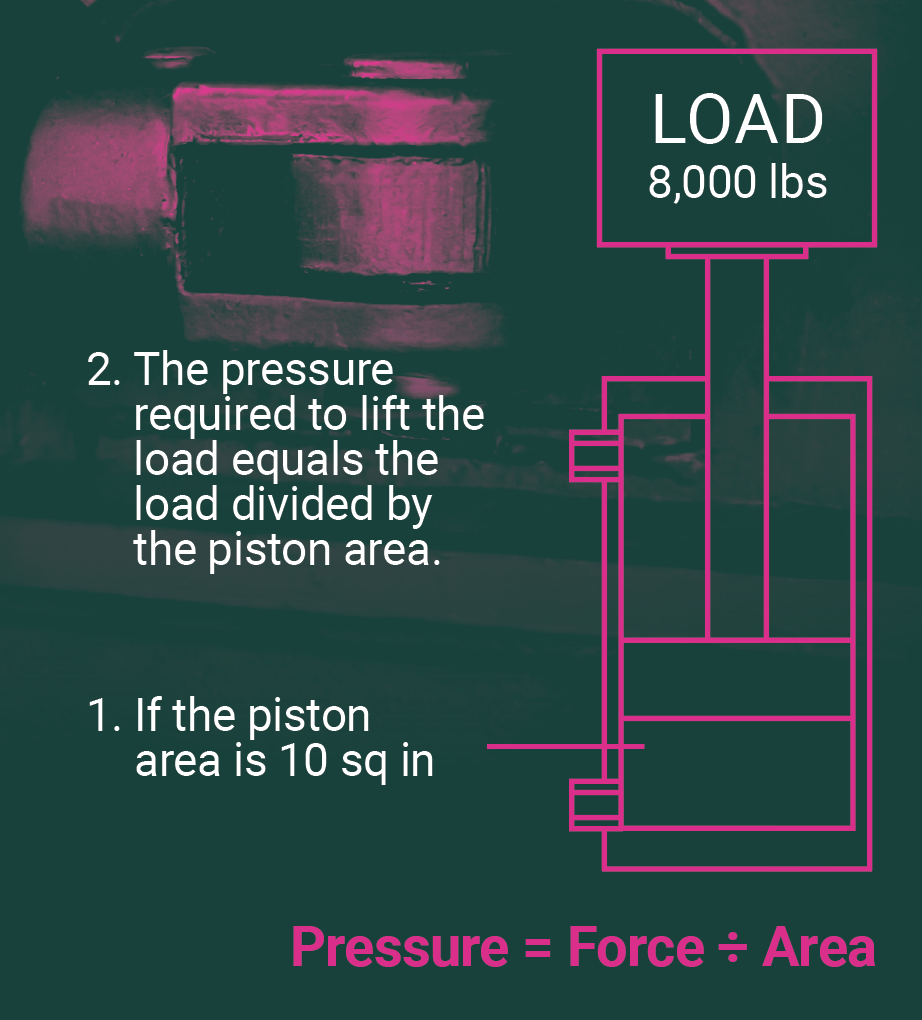
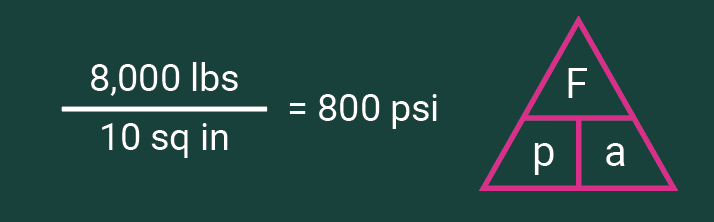
Pascal’s Law is the foundational relationship between force, pressure, and area when working with a confined fluid. In order for a cylinder to move a load or for a hydraulic motor to develop rotational force (torque), the internal area of the actuator acted upon by the force of the load will determine what the pressure must be to balance the load. If enough input power is available to provide the required flow from the positive displacement pump at the required pressure, then the cylinder or motor will move.
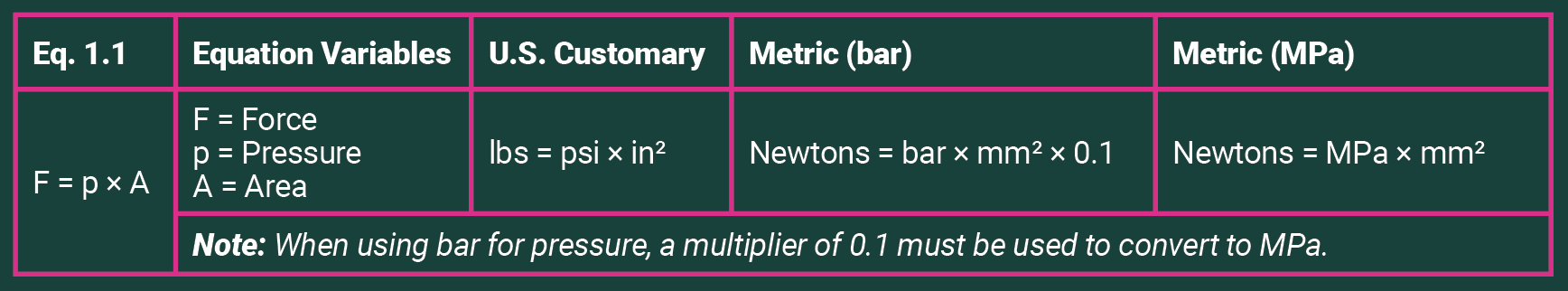
As explained in Outcome 1.2.3, Pascal’s Law is shown in Eq. 1.1 below:
Using Pascal’s Law, we can easily determine the required pressure to balance the load for any given load and cylinder dimensions.
Test Your Skills
1. If a cylinder has a 3-inch diameter bore and a 1-inch diameter rod, what is the maximum force that can be exerted when the gauge pressure is 1,000 psi?
A. 3,065 lbs.
B. 6,000 lbs.
C. 7,000 lbs.
D. 7,070 lbs.
E. 9,000 lbs.
2. If a cylinder with a 4-inch diameter bore, and a 2-inch diameter rod is mounted in a vertical position (cap-end down) and is supporting a load of 12,000 lbs, then what pressure is required on the piston (cap-end) to balance the load if the stroke of the cylinder is 20 inches?
A. 955 psi.
B. 1,500 psi.
C. 2,000 psi.
D. 2,500 psi.
E. 3,000 psi.







