What in the World Is a Megapascal

By Dan Helgerson, CFPS, CFPAI, CFPSD.

Here in the US as we move inch by inch into the metric system, we find ourselves faced with some obstacles. It is much like learning a new language.

When my son was in High School, he began to study Spanish. He progressed rather quickly and outpaced the available curriculum. In his senior year, he was given the opportunity to do a “self-study” program which he tackled with enthusiasm. He began reading novels and other literature written in Spanish. One day he walked into my study and announced that he had just realized that he was reading without translating. He was now able to think in Spanish!

Many of us who are making the effort to adopt the metric units are still struggling with having to “translate.” If we are given an equation with metric units, we first convert the units to the equivalent US Customary values, find the answer using our memorized equations, and then convert the answer back into its metric equivalent. We are proud that we have memorized the approximate equivalent US values for the metric units, but that is not quite the same as not having to translate.

There are some concepts within the metric system that help make it seem like a foreign language. For example, there is no unit of weight in the ISO metric system.

Hold on a minute! How can you say there are no units of weight in the ISO metric system? Everybody knows that a kilogram (kg) weighs 2.207 pounds.

Well, actually, no. A kg is a unit of mass, not of weight. It happens that a kg of anything on the earth at sea level will be pulled by gravity with a force of about 2.207 pounds. But that same kg at the top of Mt. Everest would have less downward force. A kg does not have an inherent weight or force. Any force involved is relative to its acceleration.

When it comes to fluid power, there is another metric unit that throws us a curve. It is the Pascal (Pa) as a unit of pressure. Units of pressure (U_p) are determined by dividing a unit force (U_F) by a unit area (U_A). In the US Customary system an example would be pound per square inch (lb./in², psi). But in the metric system, we are given the name of the man who came up with the concept. The man was Blaise Pascal, and the pressure unit is the Pascal (Pa). But what is a Pascal U_p? Well, it must be some version of U_F/U_A, but what force and what area? 

The U_F is the Newton (N), and the U_A is the square meter (m2), So, the Pa is N/m2. 

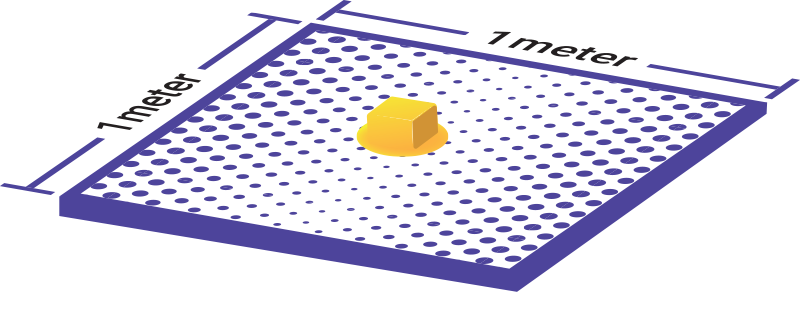
Here is a visual aid that may be of help. If you were to take a stick of butter, cut off about 10%, and then spread the rest evenly over a square meter (m²), it would have a downward force of 1 N. As you can see, the Pa is an exceedingly small amount of pressure. It is so small that the industry decided to use the Megapascal (MPa) as the preferred unit of pressure. 

The prefix “Mega” means a million. So, an MPa equals a million Newtons pressing on one m2. 

Let’s break this down a little further. A millimeter (mm) is 1/1000th of a meter (milli stands for 1/1000th). A m2 equals 1,000 mm × 1,000 mm or 1,000,000 mm2. Bear with me now. If 1 MPa equals 1,000,000 Newtons pressing on 1,000,000 mm2, then a pressure of 1 MPa equals 1 N acting on 1 mm2. 

MPa = N/mm2

When we realize that MPa = N/mm², we can see why the equation F = p × A uses, and must use, MPa as the metric U_p.  

That brings us to another issue; this U_p called the bar. Now, I don’t know who started it, so, I don’t know who to blame, but somewhere along the way, this illegitimate unit of pressure insidiously crept into the fluid power vocabulary. The bar is not a Pa, a kPa, an MPa, or even a psi. It is somewhere between a kPa and an MPa.

So, why is it called bar? The bar derives its name from the barometer. In 1665, Robert Boyle wanted to give a name to an instrument that had been invented years earlier by Evangelista Toricelli. The instrument was used for measuring the weight of air. Boyle joined together two Greek words to coin the term barometer, which means “weight measuring.” It was not until around 1900 that Vilhelm Bjerknes, the founder of the modern practice of weather forecasting, used the term bar as a unit of pressure. His barometer had a reading of 100,000 Pa at his research facility, and he considered this to be one atmosphere and called it one bar, short for barometer. However, atmospheric pressure is defined as the U_F per U_A exerted by air at sea level. His lab was 111 meters (364 feet) above sea level. So, his bar is close to, but not equal to, one atmosphere which is 101,352 Pa. But somehow, this measurement became thought of as a comfortable metric unit of pressure (U_p) and is currently (and incorrectly) used, even in our most prestigious manuals and reference material.

So, what’s so bad about the bar? We stick it in our equations, and, with a little modification, we get the correct answers.

Probably, the most important equation in fluid power is F = p × A where F = U_F, p = U_p and A = U_A. 

In the metric system, the U_F is the Newton. Those of us who are addicted to working with the bar must do a conversion to get the Newtons. For example, given a piston with a diameter of 100 mm and an available pressure of 200 bar, what would be the force in Newtons? The area of a 100 mm piston is 7,854 mm². Multiplying that by 200 bar, we get 1,570,800 units. But units of what? Minni Newtons, Macro-Newtons, fig Newtons? Hmmmm…

We can check our math by getting back into our comfort zone, solving for pounds, and then converting to Newtons. 100 mm / 25.4 = 3.937. Square this and multiply by 0.7854 and we find the area of the bore is 12.174 in². This times 2,900 psi (200 bar) = 35,303.8 pounds. To convert that to Newtons, we divide by about 0.225 and get about 156,905 Newtons. So, with the rounding, we know our target answer is in the ballpark of 157,000 Newtons. When we compare the numbers, we see that our answer using mm² and bar is off by a factor of 10, so we must adjust our equation.

What if we change the mm to cm? That might help. 100 mm equals 10 cm, so my bore area will be 10 cm squared times 0.7854 or 78.54 cm². Multiplying 78.54 cm² × 200 bar gives us 15,708 units of something but still off by a factor of 10, just in the other direction.

It seems that, to get to where we want to be, we must divide something by 10 if we really want to keep the bar.

Dividing by 10 we find ((100 mm)2/10) × 0.7854 × 200 bar = 157,080 N or (100 mm)2 × 0.7854 × 200 bar / 10 = 157,080 N

Great! We now have the answer we were looking for and were able to use the bar. Success!

But wait a second. Isn’t 200 bar / 10 equal to 20 MPa? So, (100 mm)2 × 0.7854 × 20 MPa = 157,080 N

Am I saying that, if we had just learned the equation F = p × A where F is in Newtons, p is in MPa, and A is in mm², we could have solved the problem without modification.

Yes!

Or how about the equation for Torque?

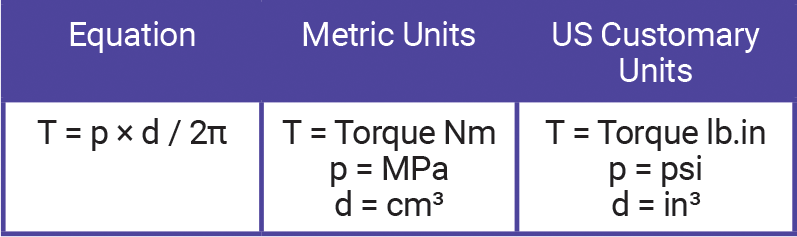
It works just fine in US Customary terms with T being torque in lb_f.in., p being psi, d being in³ displacement, and 2π being, well, 2π (6.2832). So, what if someone comes along and tells you that the metric equation must be different? It must be T = p × d / 20π. This is where T is torque in Nm, p is pressure in bar, and d is displacement in cm³. Using bar instead of MPa, the initial equation is off by a factor of 10. 2π must be multiplied by 10 to accommodate the bad bar habit. 

The next equation is for determining power. 

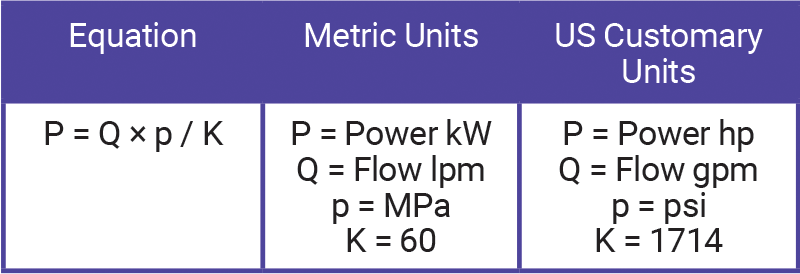
Once again, if we were to substitute bar for MPa we would have to add an additional set of units having the “K” factor be 600. Wouldn’t it be better to have a single equation each for Force, Torque, and Power that works with both metric and Imperial?

It is time to push away from the bar. After all, we may need to drive home.