Use It or Lose It

We are planning on making a system more efficient here at the steel mill. Two overdesigned hydraulic power units are each operating continuously 24/7. Each one would be capable of performing both jobs on its own, and we are thinking of consolidating the two into one system. There are some obvious environmental and energy benefits to doing this, but it requires moving us out of our comfort zone and rethinking the way we do things. In order to make the change, we will have to alter the sequence of events in our process. This required a detailed time study, partly to be certain that it can be done, and partly to assure those responsible for production that the new procedure will not interfere with productivity. In doing the time study, we found that even consolidating the power units gives us a dwell time of more than 50%. In other words, for every hour the power unit is running, the motor/pump is in a stand-by mode for over one half hour. Technically, we could use a power unit with half the capacity and run it at full load continually, storing the energy during the dwell times.

The situation seems to cry out for an accumulator, and I’m watching some of you reach for your pens so you can jot down my e-mail address to offer us your services. I appreciate that, but it is not that easy. We are trying to save energy and reduce complexity. The various functions use pressures ranging from 40 bar to 125 bar, and there is a requirement that our pump pressure not exceed 140 bar.

If this were a pneumatic system, it would be no big deal. We would automatically think of sizing the compressor for the average flow and then put on a receiver to store the energy during the dwell times. Because of the way we store and release compressed air, we would use only the air molecules necessary for the job. Even if we store the energy at substantially higher pressures than required for the loads, the proper use of pressure regulators would mitigate the energy loss.

But this is a hydraulic system. If we store the energy in a common gas accumulator with a maximum pressure of 140 bar and a minimum pressure of 125 bar, we would need a gas volume 15 times greater than the available fluid volume. When the load requirement is only 40 bar, the 100-bar differential would be lost in heat. If we were to use weighted accumulators, we would reduce the accumulator volume, but we would still have to deal with the varying pressure requirements and the 100-bar pressure differential lost as heat during the low-pressure portions of the cycle.

This brings us to the title of the article. The most efficient fluid power system is the one where the flow and pressure exactly match the load requirements. When we store hydraulic fluid in an accumulator, whether gas, spring, or weighted, almost by definition, we will waste some energy as we release it, restricting either the flow or the pressure or both. When we use a pressure-compensated or load-sensing pump, our pressure setting or our differential pressure setting will be higher than the load requirement and we will waste energy. Our flow will match the speed, but our pressure will be higher than the load requires. To be efficient, we need to use all the stored or induced energy for useful work. What we do not use for useful work we will lose in Btu’s. Use it or lose it.

A couple of articles ago, we discussed the fact that we have an advantage when it comes to alternative energy systems because we have the ability to store energy in ways that no mechanical system can match. Our weakness is in the wasteful way we typically release that energy.

We put energy into a fluid when we push it against a resistance. That resistance could be a load we are trying to move, a restriction such as a flow control valve, or, if we are storing the fluid for future use, the resistive load of an accumulator. When we take a volume of fluid out of storage, it has a potential energy based on the pressure under which it was stored. When we use that volume of fluid to do some work, we direct the potential energy to the load.  Because of the relative incompressibility of liquid, the only variable in the energy equation is the pressure. If the load requires more pressure than is available, no work is done. If the load requires less pressure than is available, work is done and the remaining energy is lost in heat.

What we should try to do is use all the energy in the fluid to do useful work regardless of the flow and pressure requirements of the load. If we can take all the energy in a stream of fluid and do useful work regardless of the initial pressure, we will be able to make some very energy-efficient systems.

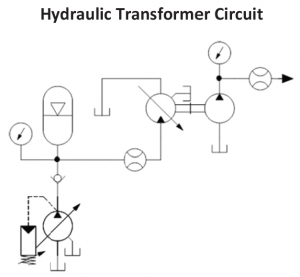
I am suggesting, for want of a better term, a variable hydraulic transformer that matches the available pressure and flow to the required pressure and flow. It is simply a variable displacement hydraulic motor coupled to a fixed displacement hydraulic pump as in the circuits below.

Some of you are going to want to stop reading right now because it is going to get technical, but give it a shot and at least try to get the concept. Get out your calculators and check my math to see if it all makes sense. Forget the fudge factor, reject the rule of thumb, and shun the shortcut. Do the math.

This is how it would work: we will have the variable displacement of the motor controlled electronically. A flow transmitter would be placed in the pressure line from the pump, and its setting is what would determine the displacement of the motor. No matter what the load pressure was, the motor would be commanded to drive the pump at an RPM that would produce the required flow. The motor must develop enough torque to drive the pump, and so the motor output torque will be the same as the pump input torque. Torque is a factor of the pressure and the displacement. If we call the available pressure from the accumulator P₁, the motor displacement D₁, the load pressure P₂, and the pump displacement D₂, then P₁ x D₁ = P₂ x D₂. For any given flow and pressure requirement from the pump, there will be a discreet displacement required from the motor based on the available pressure.

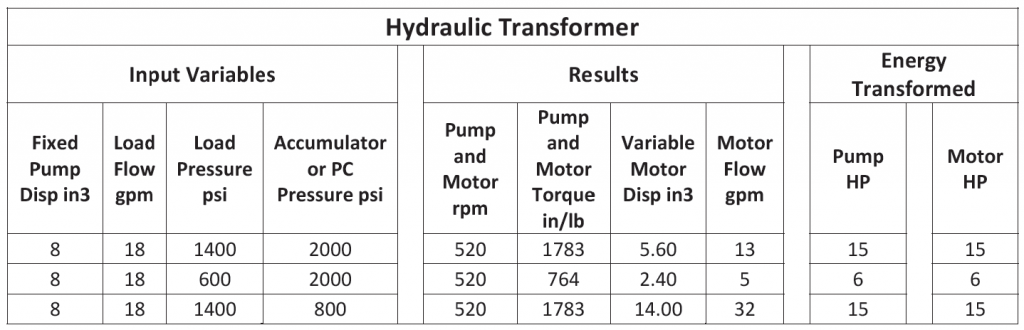
Let’s walk through an example. We have a pump with a displacement of 8³ inches, and we are asking it for a flow of 18 gpm at a resistive pressure of 1400 psi. The energy source for the system is an accumulator loaded to 2000 psi. We know that torque equals the pressure times the displacement divided by 2π (T = P x D_isp / 6.2832) and that the flow equals the displacement times the RPM divided by the number of cubic inches in a gallon (Flow = RPM x D_isp / 231). Doing the math, 18 = RPM x 8 / 231 and solving for RPM, our pump will have to rotate at about 520 RPM and will need an input torque of… anybody what to jump in here?? Ok, you with the IFPS shirt (available at the IFPS Store), what have you got? That’s right! 1400 x 8 / 6.2832 = 1,783 in/lb of torque.

So, what will be the motor torque? Right, again! The motor torque has to be the same as the pump torque so it will be… 1,783 in/lb. What will be the displacement of the motor? Remember the formula: 1,783 = 2000 x D / 6.2832 and solving for D we find the motor displacement to be 5.6³ in. Some of you may also have noticed that the ratio of the displacements of the pump and motor is the same as the ratio of the load and available pressures.

However, the controlling flow transmitter is not looking at the pressure, only the flow. If the flow begins to exceed the command setting, the control will cause the motor displacement to decrease to where the motor is just able to maintain the necessary torque to maintain speed. If the flow drops below the command setting, the control will cause the motor displacement to increase, maintaining speed. As the accumulator drains down or as the load increases, the flow transmitter will direct the motor displacement to increase to maintain speed. The motor displacement will always try to match the available pressure to the torque requirement of the system. It will also become the flow control matching the motor RPM to the pump flow. All the available stored energy will be used for work, and the only losses will be from the relative efficiencies of the components.

In the table and circuit below, you will see three different pressure scenarios. The first is what is described above. The next two show what happens when the input or output pressures change. Notice that the pump and motor HP is the same for each scenario.

Use it or lose it? Let’s use it.

• circuit
• dan helgerson
• efficiency
• energy
• fluid power
• hydraulic